Analysis of a clarinet sound
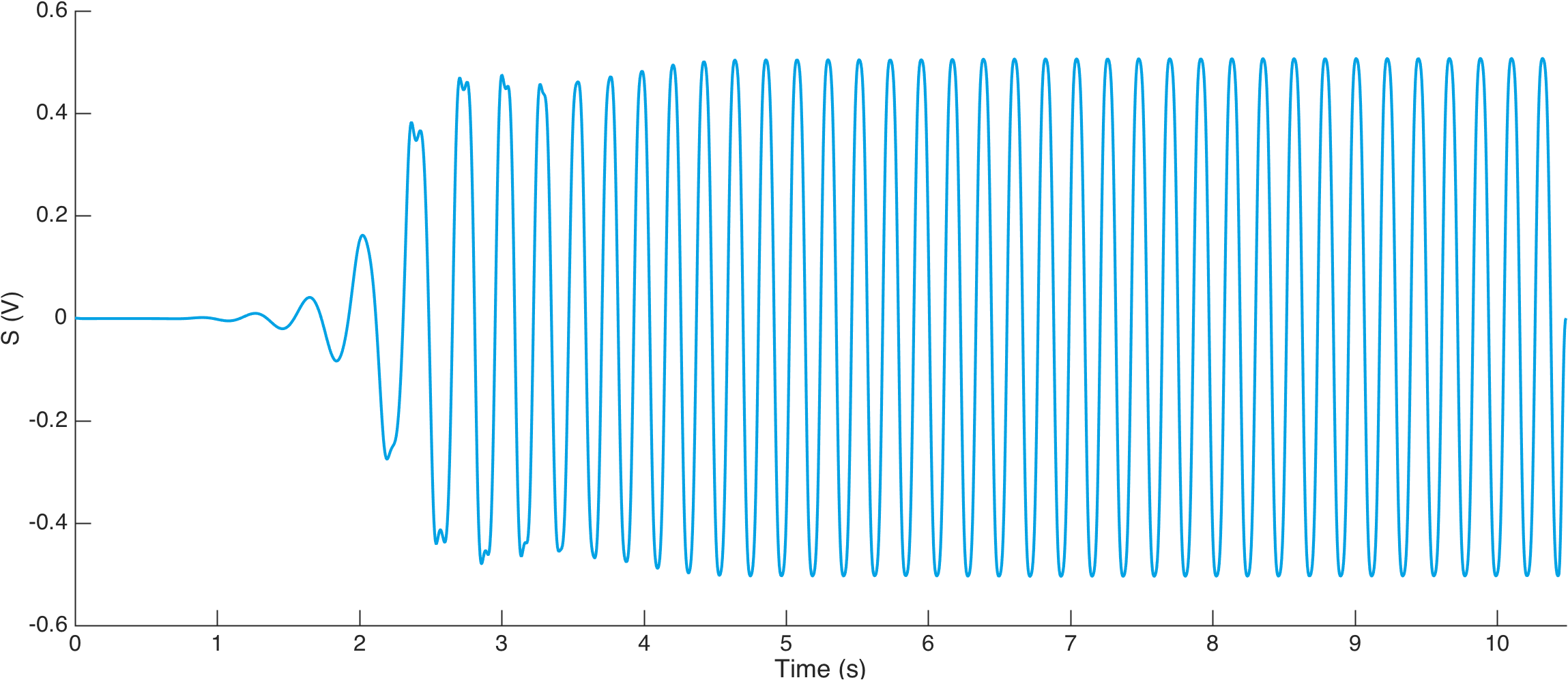
The following instance perfectly illustrates the possibilities offered by wavelet time-frequency analysis to extract main features hidden in weak signal components and whose Fourier analysis can hardly reveal. The signal considered here is a digital record of a clarinet sound that was rescaled in time and whose time response is populated with repeated oscillations likely to be related to a stationnary frequency. In other words, the sound happens to be a single note of music that was played during quite a long period of time.
A display of the Fourier power spectrum of the clarinet signal clearly indicates the occurrence of two frequency peaks
A display of the Fourier power spectrum of the clarinet signal clearly indicates the occurrence of two frequency peaks
- first peak f0 = 4,9 Hz stands for the fundamental frequency of the note
- second peak f3 = 13,7 Hz is the 3rd harmonic of the note
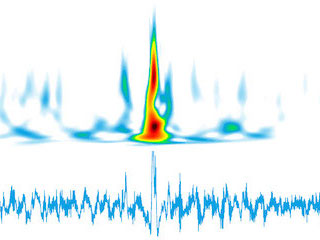
Wavelet scalogram of clarinet sound
The scalogram of clarinet sound computed with the Meyer wavelet permits to explore the sound dynamics with a brand new glance. Sound energy happens to be mainly localized in two clusters within different frequency bands
- a first cluster in red arises at the beginning of the note in a time-frequency area neighbouring f0=4,9 Hz and lives as as long as the note is played
- a second cluster in blue starts one second after the note in a frequency band surrounding f3=13,7 Hz and its energy rapidly vanishes after time 3,5 seconds
Instantaneous frequencies of clarinet sound
A crazy climber algorithm was used to calculate the ridges of wavelet clusters that concentrate most of the energy of clusters and that permit to follow the time-evolution of instantaneous frequencies of the sound components
- frequency of the ridge of the first cluster starts above 2,2 Hz at the beginning of sound then gradually increases similarly like a chirp signal before stabilizing on the fundamental frequency f0=4,9 Hz of the note
- the ridge of the second cluster starts a little bit later and climbs from above 8Hz till frequency f3=13,7 Hz before almost vanishing due to a lack of energy
 English (UK)
English (UK) Français (FR)
Français (FR)